Internal Model Control (IMC) is a new control strategy based on process mathematical model for controller design.
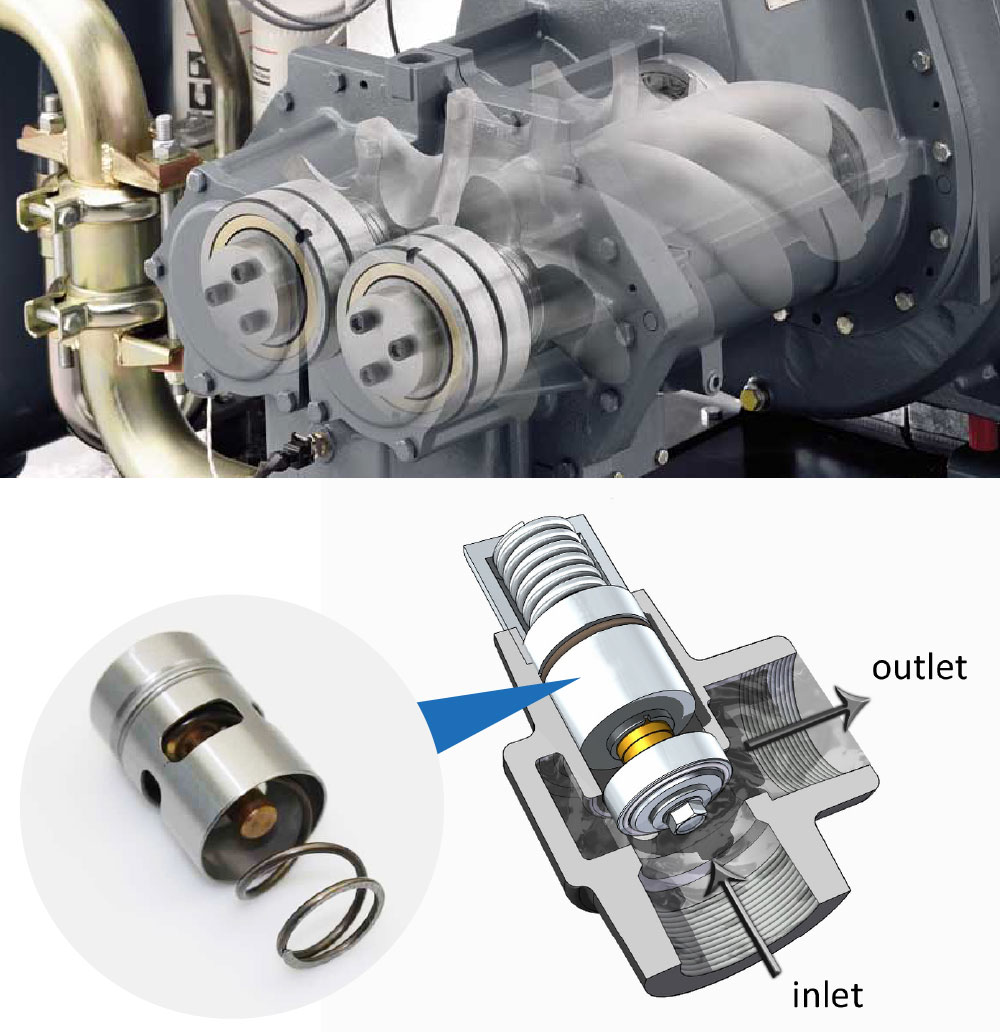
Because of its simple design, good control performance and superiority in system analysis, IMC is not only a practical advanced control algorithm, but also an important basis for studying model-based control strategies such as control, and a powerful tool for improving the design level of conventional control systems. The internal model control theory proposed by Carcia and Morari in 1997 introduced an internal model into the system, which made the design of the controller easier. As a practical control method, IMC is characterized by simple structure, intuitive design and clear adjustment policy, especially for the improvement of robustness and immunity and the control of large time-delay systems.
Since its emergence, it has been widely used not only in slow response process control, but also in fast response motor control, which has better performance than traditional PID control.
The basic internal model controller structure is shown in Figure 1. Because this structure includes not only controller C (s), but also process model G (s), internal model control is named. This simple IMC structure has been extended to MIMO systems and non-linear processes, laying a solid foundation for internal model control.
In this way, the idea of designing controller by inversion of process dynamic model has been realized in engineering.
If the model is accurate, i.e. without external disturbance, i.
e. d(s) = 0, then the input of the model is equal to the output y of the process, and the feedback signal is zero. In this way, the internal model control system has an open-loop structure under the condition of model uncertainty and unknown input. This clearly shows that for open-loop processes, the purpose of feedback is to overcome the uncertainty of the process. That is to say, if the process and process input are completely clear, only feedforward (open loop) control is needed, and no feedback (closed loop) control is needed. In industrial process control, overcoming disturbance is the main task of control system, and the uncertainty of model is unavoidable. At this point, in the IMC structure shown in Figure 1, the feedback signal reflects the uncertainties and disturbances of the process model, thus constituting a closed-loop control structure. First, duality stability.
Assuming that the model is accurate, that is to say, the necessary and sufficient condition for internal stability of IMC system is that both process G (s) and controller C (s) are stable. Second, the ideal controller characteristics. Thirdly, controller design. The design of internal model controller is divided into two steps. Step 1, thermostatic element decomposition of process model. Here, it is an all-pass filter transfer function, which contains all delays and right half-plane zeros. It is a transfer function with minimum phase characteristics. Step 2, IMC controller design. In order to ensure the stability and robustness of the system, a filter must be added to the minimum phase when designing the IMC controller. In the formula, f (s) is a low pass filter. One of the purposes of selecting f (s) is to make GC (s) rational. A is the filter parameter, which is the only design parameter of IMC. Higher-order processes with right half-plane (RHP) zeros. Here N (s) and D (s) are polynomials of S. The inverse of N (s) is unstable when the N (s) term of equation (10) contains polynomials of form (-Ts 1) or higher order containing zeros of the right half plane. In this case, the internal model controller can not be constructed according to the open form given in formula (8), which is a simple first order plus pure delay design method. For this process, an IMC controller is designed to optimize the integral square error (ISE) by inversing some models containing zeros of the left half plane and adding poles to the mirror mapping of the imaginary axis with zeros of the right half plane. It can be seen from equation (11) that the system is a process with odd right half zeros.

At this time, the initial response of the process to the step is opposite to the final steady state of the response, and the process shows the characteristics of inverse response. Fig. 3 shows the system response when the parameters of the controller are 0.2, 0.5 and 1, respectively. The simulation results show that the ISE value of 0.
2 is the smallest and the response speed is the fastest, but the initial overshoot is also the largest. The response speed of about 0.7 seconds is obtained at the cost of 0.
2 larger than the initial overshoot of 0.5. The effect is still very ideal for the process with large time delay. Therefore, the controller design based on ISE optimization standard is most suitable for process control with large time delay, and can effectively speed up the response of the system. In this paper, an internal model controller is designed for high-order processes with zeros.
The filter parameters are selected based on ISE optimization criteria. The response time of large-time-delay processes is effectively accelerated. The simulation results of MATLAB show that the internal model controller has a good control effect on large-time-delay processes.
